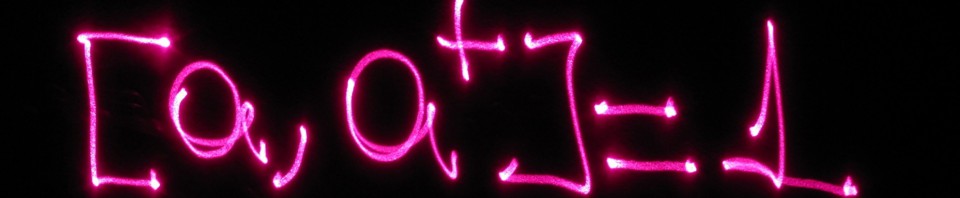We have provided the first demonstration of a general orthogonalization procedure that, starting from an arbitrary continuous-variable quantum state and from limited information about the state itself, can generate an orthogonal state and arbitrary coherent superpositions of the two.
We expect this achievement to substantially advance quantum state engineering and information processing with new tools: implementation of a quasi-universal NOT operation and generation of arbitrary continuous-variable qubits.
Besides possible applications, this method has a general fundamental appeal as a sort of Schrödinger’s box able to convert any input state into a quantum superposition.
See ArXiV:1407.6644v1